

They develop an awareness of chance by playing with materials or objects that involve cause and effect (actions that will happen) and playing games where the outcome is unpredictable. Students participate in class activities that explore object, events and displaying information. Students respond to specific instructions relating to manipulating the movement and location of self and objects. They can match identical familiar three-dimensional shapes that are ‘the same’. They demonstrate an awareness of object permanence by searching for objects that have been hidden and participate in class activities that explore three-dimensional objects. They explore routine events and show an awareness of time and daily routines by responding to a routine signal from the teacher. Students demonstrate beginning understanding of basic measurement concepts such as ‘long or short’, ‘heavy or light’. Students participate in everyday activities that explore measurement and use measurement attributes in practical situations. They manipulate objects and build a tall tower by using ‘more’ blocks and take blocks away from a tower to make the tower ‘less’ tall.
Students identify ‘one’ and ‘lots’ of objects and show an understanding of ‘more’ in familiar situations. Students participate in everyday activities that involve numbers and counting, comparing groups of objects, and pattern activities. They respond to major changes to regular games and activities associated with chance, surprise and predictability, such as moving a switch to activate a toy. Students begin to display a similar and predictable reaction to regular events. Students observe objects and events within their daily life. Students explore space by moving and changing position and location, and respond to changes in position. Students explore and respond to objects of varying textures, colours, sizes and shapes. Students respond to a signal from a timer, used to indicate the end of an activity. They show an awareness of time and daily routine by responding to a signal from the teacher, and items being brought out or removed. Students explore objects of varying weights, lengths, capacities and materials. They react and respond to objects and experience measurement attributes in practical situations. Students observe and explore objects within daily life. Students participate in making piles, groups or bundles of familiar everyday objects and respond to objects being put together and taken apart. They experience and respond to ‘one for you, one for me’, ‘gone’, ‘no more left’ and ‘give me more’. Students demonstrate awareness of counting by responding to number rhymes, songs, stories and finger games.

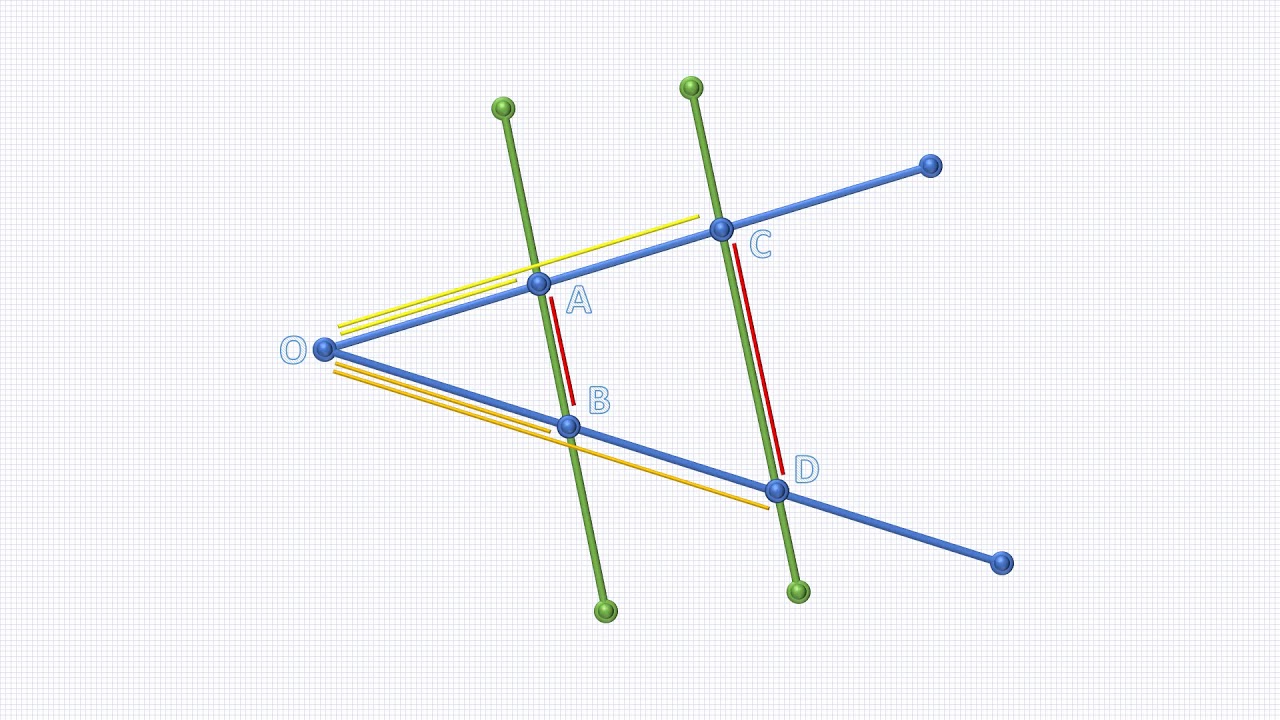
They begin to respond to numbers in everyday experiences. Angles that are between 90° and 180° (larger than right angles and less than 180°) are called obtuse angles.Students observe the use of number within their daily life. A corner mark is often used to denote a right angle, as shown in right angle \(\ D C B\) below.Īngles that are between 0° and 90° (smaller than right angles) are called acute angles. Rectangles contain exactly four right angles. This represents exactly one-quarter of the way around a circle. (In skateboarding and basketball, “doing a 360" refers to jumping and doing one complete body rotation.)Ī right angle is any degree that measures exactly 90°. For example, a circle is defined as having 360°. The length of the hands does not impact the angle that is made by the hands.Īn angle is measured in degrees, represented by the degree symbol, which is a small circle at the upper right of a number. As time passes, the hands rotate around the fixed point, making larger and smaller angles as they go. The minute and hour hands are both fixed at a point in the middle of the clock. Drawings of angles will often include an arc (as shown above) to help the reader identify the correct ‘side’ of the angle. The length of the rays has nothing to do with the size of the angle itself. When people talk about the “size” of an angle, they are referring to the arc between the two rays. Sometimes angles are very narrow sometimes they are very wide. Notice that the label of each angle is written “point-vertex-point,” and the geometric notation is in the form \(\ \angle A B C\). The image below shows a few angles on a plane. When you are naming angles, be careful to include the vertex (here, point \(\ B\)) as the middle letter. This angle can also be called \(\ \angle A B C\), \(\ \angle C B A\), or simply \(\ \angle B\). The common endpoint of the angle is called the vertex. For example, two rays with a common endpoint make up an angle. Lines, line segments, points, and rays are the building blocks of other figures.


 0 kommentar(er)
0 kommentar(er)
